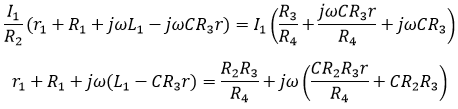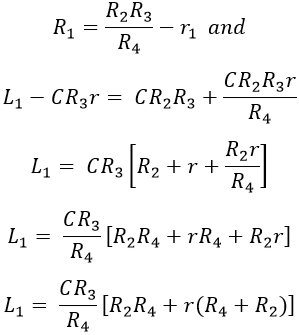Anderson's Bridge - Circuit Construction, Equation, Phasor Diagram & Advantages

The Anderson's bridge is an alternating current bridge, which is used to find the self-inductance of the circuit. Actually, this bridge is the modified form of Maxwell's inductance capacitance bridge for the measurement of self-inductance in terms of the standard capacitor of low Q coils (Q < 1). The bridge uses a fixed capacitor instead of a variable capacitor as seen in Maxwell's bridge and hence gives more accurate results.

Construction of Anderson’s Bridge :

The bridge consists of four arms AB, AD, BC, and CD. The arm AB of the bridge consists of unknown inductance to be measured in series with a non-inductive resistance R₁ and r₁ be the resistance of the inductor. The below shows the circuit diagram of Anderson's bridge under balance condition.

The arms AD, BC, and CD consist of standard non-inductive resistances R₂, R_3, and R₄ of known value. It consists of extra junction E to which a variable resistor r is connected between E and D, a fixed capacitor between E and C, and a null indicator or detector between E and B.

Operation and Theory of Anderson’s Bridge :

Let,

• C = Standard capacitor of fixed known value
• R₂, R₃, R₄ = Standard non-inductive resistances of known values
• L₁ = Self-inductance to be measured
• r₁ = Resistance of the unknown inductor.

Under balanced condition, we have,

From equation 3, we have,

From equation 4, we have,

From equation 5, we have,

From equations 6 and 7, we have,

Equating real and imaginary parts on both sides, we get,

Therefore, the unknown value of self-inductance is,

The unknown value of resistance of the self inductor is,

Phasor Diagram of Anderson’s Bridge :

Taking current I₁ as the reference phasor. The current I₁ is passing through arm AB i.e., through non-inductive resistance R₁ and inductor L₁. Thus the total drop V₁ in arm AB is I₁ (R₁ + r₁) (which is in phase with I₁) and I₁ ωL₁.

When the bridge is balanced the same current will also pass through R₃ (in arm BC) i.e., I₁ = I₃. Thus the drop I₃ R₃ (i.e., V₃) lies along the phasor I₁. Also, we know that the potential between junctions BC will be equal to the potential between junctions EC. Hence V₃ = I_c/ωC and the drop I_c r in the resistor r will lie along with I_c.

When the potential across the detector is zero (balanced condition) V₄ will be equal to V_EC. Thus V₄ is nothing but the sum of drop, in resistor r, and V_EC or V₃. Adding the drop I_c r to the V₃ we get V₄ (i.e., drop I₄ R₄ in arm CD), also the current I₄ lies along with V₄.

Also, when the bridge is at balance condition, the current I₂ is the sum of the currents I_c and I₄, also the drop V₂ (i.e., I₂ R₂) in arm AD due to I₂ lies along with it. Therefore, the resultant of the phasors V₁ and V₃ or V₂ and V₄ gives supply voltage V.

Advantages of Anderson's Bridge :

• The problem of sliding balance condition normally faced with low Q coils is overcome in Anderson's bridge. This is because both the variable resistances to be adjusted are independent of each other. Hence, the balance can be obtained easily.
• Instead of a variable capacitor, a fixed capacitor can be used. This makes the bridge cheaper than Maxwell's bridge.
• Determination of unknown capacitance in terms of known inductance is also possible.
• The expression for self-inductance of the coil does not change even with the use of an imperfect capacitor (i.e., the capacitor with dielectric loss). Instead, only the value of coil resistance is affected.
• For more precise measurements, a second balance is obtained by short-circuiting the coil, and the inductance of coil leads is calculated. Finally, the actual self-inductance of the coil is obtained by subtracting the values of inductances obtained in both the measurement cases.

Disadvantages of Anderson's Bridge :

• The Anderson's bridge (which is a modified form of Maxwell's bridge) is more complex in terms of circuit connections and computations when compared to Maxwell's bridge due to the increase in the number of components used in the circuit.
• The balance equation calculations are also complicated than Maxwell's bridge calculations.
• Shielding of the bridge circuit is difficult as an additional junction point is introduced in the circuit.